G.0816
Braking
There is a well-known saying among automobile engineers that the brakes of a car take out all the energy that the fuel puts in. In other words, if you are designing a powerful car, you will make sure that the brakes match the performance of the engine so they can bring the vehicle to a halt quickly enough to give protection to the occupants and other road users as well. Does the same principle apply to all modes of transport? Common sense suggests that it should, but the picture is a mixed one. Many railway trains have complex and sophisticated braking systems, especially intercity passenger sets, some of which travel three times faster than a typical family saloon. On the other hand, the brakes are rarely used to their full capacity, and if they were, passengers would complain. The case for sea-going craft is different again: there’s no equivalent of the disk brake for stopping a ship, at least not a large one like an oil tanker. Stops have to be planned several minutes in advance and in an emergency, the helmsman can only steer out of trouble [1]. The situation for aircraft is more complicated. Once aloft an aircraft can’t stop at all, and even flying slowly is not a good idea. On the runway, however, an aircraft must be capable of aborting a take-off if something goes wrong, and its brakes must cope with very high loads. Our aim in this section is to compare the demands made on the braking system by different types of vehicle ranging from bicycles to jumbo jets.
Energy dissipation in braking
To design an effective braking system you need to know how it will be used, and in particular, the maximum energy flows it must handle. The kinetic energy embodied in a vehicle of mass \(M\) travelling at speed \(V\) is \(\frac{1}{2}MV^{2}\), and to make the vehicle stop, all of this energy must be removed in a very short time. But there is another kind of energy to be taken into account. When a vehicle coasts downhill, it is losing potential energy and will continue to do so until it reaches level ground. If the total change in height is \(h\), the change in potential energy is \(Mgh\), and this must be dissipated continually throughout the descent if the vehicle’s speed is to be kept under control. Heavy braking over long periods can heat up the brakes until they no longer perform properly, and it is for this reason that long downhill grades present a further design challenge for road vehicles (see Section C0816) and railway trains. Aircraft pilots manage even longer descents by other means which we’ll touch on elsewhere.
Friction
There are three kinds of brake in common use: the friction brake, the electromagnetic brake, and the aerodynamic brake, each of which dissipates energy in a different way. Most friction brakes work by gripping a wheel or an axle to slow down the rate of rotation. On each wheel of a car, for example, a pad presses against the metal surface of a disk or drum. The resulting torque doesn’t slow the vehicle directly. The force that actually slows the vehicle down comes from the road surface acting on the tyre tread (figure 1). So however powerful the brakes themselves might be, the braking force is limited by the coefficient of friction between the tyre and the road. A similar limitation applies to rail vehicles, and since steel railway wheels running on steel rails have a relatively low coefficient of friction, trains can’t stop very quickly and need a relatively large gap between them to avoid collisions.
Figure 1

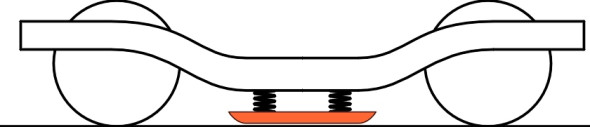
However, there is another way to stop a railway vehicle: instead of gripping the wheels, the brake pads can grip the track instead. This is the principle behind the emergency brakes used on modern trams. Longitudinal steel shoes are mounted on either side of each bogie, and in normal operation are held in a raised position just above the track (figure 2). They are activated by electromagnets whose magnetic field attracts them towards the rails. When applied, they clamp themselves to the rails and bring the tram to a halt.
Figure 2
Non-friction methods
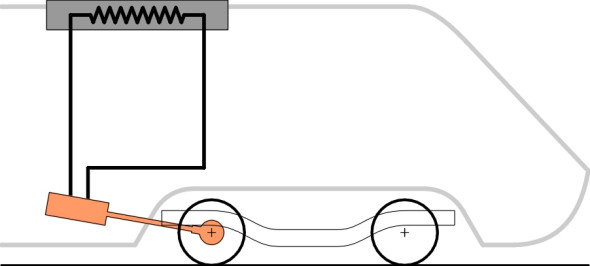
All friction brakes have an abrasive action that removes material from the surfaces in contact, and during the 1970s, an express loco would wear out its brake shoes in about a week. For braking mechanisms that belong to our second category, ‘rheostatic’ brakes, there is no frictional contact at all. Rheostatic brakes are designed for use on electrically-powered vehicles. They work by reversing the mode of operation of the motor, so that it becomes a generator that is powered by the wheels. Since mechanical work has to be put into a generator to create a current, the motor then acts as a brake. The current is fed to resistors on the train roof, where the energy is dissipated as heat, or in some cases, returned to the overhead electrical supply (a schematic diagram is shown in figure 3). Originally, rheostatic brakes were introduced on diesel-electric and electric locomotives to reduce the load on their friction brakes and hence reduce wear. Having proved themselves as back-up devices, rheostatic brakes then took over as the principal system on British locos, where they were designed to dissipate energy at a rate of 2 MW [5]. Apart from the question of wear and maintenance, they were quieter than friction brakes and the braking effort was easier to control.
Figure 3
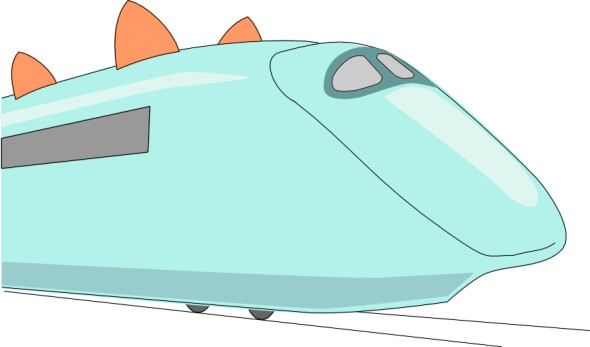
Our third and last category relates to aerodynamic devices that grip neither the wheel nor the track, but the air through which the vehicle is moving. Since air resistance increases as the square of speed, they can be effective on vehicles travelling over 100 km/h. Moreover, they don’t overheat and they don’t wear out. Air brakes have been used in the past on sports racing cars, notably by the Mercedes Benz team at Le Mans during the 1950s, when drum brake technology couldn’t keep pace with increasing speed in duration events. Today, it is train manufacturers who are reviving the air brake as an auxiliary system for high-speed running. Built in 2005 by the East Japan Railway Company, the prototype tilting Japanese bullet trains ‘Streamline’ and ‘Arrowline’ are designed to run at 360 km/h (225 mph). Since the coefficient of friction between wheels and track is only around 0.25, aerodynamic assistance can be invaluable for emergency braking at this speed. The brakes are flat fins aligned at right-angles to the direction of motion (figure 4). Normally retracted inside the body shell, they are extended by hydraulic rams for emergency braking [10].
Figure 4
Some performance comparisons
Brake materials
Friction brakes rely on sophisticated materials that can transmit frictional forces and conduct heat away from the contact area without melting or changing shape. Many different combinations have been tried, and the figures set out in table 1 illustrate the characteristics of different materials used on braking systems developed at different times during the last few decades (the coefficients of friction in the last row were culled from internet sites advertising motor racing and aircraft products).
| \(\) | Material | Coefficient of friction | Lifetime |
| Automobiles [7] | Resin-impregnated fibre pad on cast iron disk | 0.3 – 0.5 | Several years depending on use |
| Railway vehicles [3] | Cast iron shoe on steel wheel rim | 0.2 | 1 year |
| Aircraft landing gear [4] | Sintered metal | 0.3 | Several dozen applications |
| Aircraft landing gear and high-performance cars | Carbon fibre in ceramic matrix | 0.4 - 0.6 | Depends on usage |
Notice that the coefficient of friction \(\mu_{s}\) rarely exceeds 0.4 (we are referring to sliding friction here, hence the subscript \(s\)). One might have expected the values to be higher in view of the need to stop the vehicle as quickly as possible. But the frictional force between brake pad and disk doesn’t just depend on \(\mu_{s}\); it is also proportional to the normal contact force \(N\) (see section G2016). Unlike tyres, which rely on the weight of the vehicle to supply the normal contact force, a brake pad is pressed against the disk under hydraulic pressure, which within reason can be increased to any desired level. Nowadays, high performance brake disks are made from carbon composites, typically carbon fibre in a ceramic matrix. Although fashionable for sports cars, the main advantage in comparison with metal disks is the weight saving, and the ability to operate at high temperatures. On a competition racing car, they glow red hot when the driver brakes on the approach to a bend.
Stopping distances
During the eighteenth century, a horse-drawn carriage was usually equipped with a drag shoe, a portable device like a shovel that could be wedged under the rear wheel to prevent it rotating. The coach was then supposed to slither downhill with the wheel locked [2]. But the drivers of those days saw little virtue in brakes, and rarely used them. In the 1840s, many steam locos capable of 60 mph had no brakes [11]. Since that time, governments throughout the world have introduced minimum standards for almost all types of vehicle, and later, we shall see how engineers have responded (brake technology for cars is reviewed in section C0816). For now, we’ll merely try to gauge the size of the task involved.
The criteria that a braking system must satisfy vary considerably according to the conditions under which the vehicle operates, and the forces that passengers can tolerate. Road vehicles undergo ‘near-misses’ quite frequently and must therefore be capable of an emergency stop at high rates of deceleration. Since the passengers are strapped in, a deceleration of \(0.5g\) is tolerable. On a dry surface, in theory a car can stop at \(1g\), but drivers rarely use the full braking power available even in an emergency. Air passengers are also strapped in, and are accustomed to moderately large \(g\)-forces on landing. By contrast, train passengers might be sitting, standing, or walking about on board at any time during the journey, and therefore likely to fall over if subjected to braking forces of a comparable level. The parameters of the signalling framework are fixed so that if the train is required to stop for a signal, the rate of deceleration is around \(0.05g\), although higher rates of deceleration are possible in an emergency. Trams, on the other hand, are not protected by a block signalling system, and because they share road space with private traffic, they experience sharp deceleration more frequently.
| Category | Vehicle details | Mass | Initial speed | \(g\) |
| \(\) | \(\) | (tonnes) | (km/h) | (\(\times\) 9.81 m/s2) |
| BICYCLE | \(\) | 0.09 | 32 | 0.50 |
| CAR | Typical family saloon at motorway speed | 1.5 | 113 | 0.50 |
| TRAM | Midland Metro (Birmingham, UK) [9] | 54 | 75 | 0.255 |
| RAILWAY TRAIN | TGV Paris Sud Ouest [8] | 385 | 270 | 0.20 |
| JET AIRLINER | Airbus 380 [6] | 500 | 322 | 0.285 |
| SHIP | Oil tanker | 200 000 | 25 | NA |
So taking the various constraints into account, how much stopping distance does each type of vehicle need, and how much energy must be dissipated during the process? Some notional deceleration rates are set out in table 2; they represent what is required from the braking system during an emergency stop. Also in the table are assumed figures for the vehicle mass and initial speed.
Based on the above figures, table 3 shows for each vehicle type the distance and time required to stop. The braking distances for the high speed train and for the passenger airliner are both well over a kilometre. For reasons already explained, ships need even more. Assuming a constant rate of deceleration, one can easily estimate the corresponding energy dissipation rates, which are shown in the last column. The figure of 62 MW for the Airbus A380 is equivalent to power output of a small electric power station. Each wheel on the landing gear handles a braking load equivalent to the 8 wheels of a moderately heavy truck, and multiple disks are needed to shed the heat into the surrounding air so it doesn’t set fire to the tyre.
| Category | Distance | Time | Energy dissipated | Rate of dissipation |
|---|---|---|---|---|
| \(\) | (m) | \(\) | (MJ) | (MW) |
| BICYCLE | 8 | 1.8 s | 0.003 | 0.002 |
| CAR | 100 | 6.4 s | 0.73 | 0.11 |
| TRAM | 87 | 8.3 s | 12 | 1.4 |
| RAILWAY TRAIN | 1433 | 38.2 s | 1080 | 28 |
| JET AIRLINER | 1430 | 32 s | 2000 | 62 |
| SHIP | \(\) | \(>\)12 minutes | \(\) | \(\) |
Conclusion
Whenever it stops or slows down, a high-speed vehicle sheds a great deal of energy, and the energy is usually wasted. In this sense, braking manoeuvres contribute to fuel consumption and carbon emissions. Some of them are avoidable. In an ideal world, the person in control of the vehicle would anticipate events well in advance, and instead of applying the brakes, use the various forms of resistance to motion such as rolling resistance and aerodynamic drag to slow the vehicle down. Cyclists do this unconsciously because it saves physical effort. Ship’s captains do it because there is no alternative. But on crowded urban roads, car drivers have little incentive to economise in this way. On a transport network with many junctions, the most effective way to save fuel is through co-operative movement under centralised control. Railways have been doing this for a long time, and their efficiency continues to improve with more sophisticated forms of moving block signals, but for motorists, a cooperative system is some way off.